Rotational Dynamics Concepts Page - 12

Example

Problems on collisions of rigid bodies using angular impulse relations

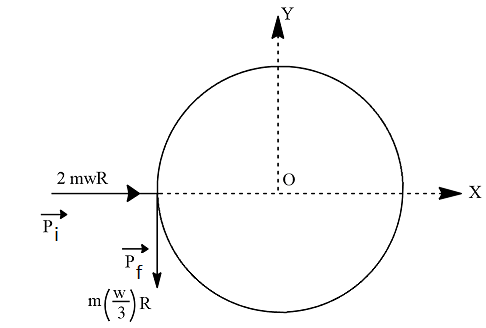
Example: A uniform disc of mass m$m$ and radius R$R$ rotates about a fixed
vertical smooth axis passing through its centre with angular velocity ω$\omega$ . A particle of same mass m$m$ and having velocity
2$2$ω$\omega$R$R$ towards centre of the disc collides with the disc
moving horizontally and sticks to its rim. Then what will be the angular velocity of the disc and the impulse imparted on the disc?

Solution:
Since the particle of mass hits the rim radially and sticks to it, there is no torque acting on the particle about the center O. Hence, angular momentum of  the system will be conserved.
L=Iω$L=I\omega$ is conserved.
Li=Lf$L_i=L_f$
⇒mR22ω=(Idisc+Iparticle)    ωf=(mR22+mR2)ωf=32mR2ωf${\displaystyle \Rightarrow \frac{m{R}^2}{2}\omega =(I_{disc}+I_{particle}){\omega }_f=({\displaystyle \frac{m{R}^2}{2}}+m{R}^2){\omega }_f={\displaystyle \frac{3}{2}}m{R}^2{\omega }_f}$
⇒ωf=ω3$\Rightarrow {\omega }_f={\displaystyle \frac{\omega }{3}}$
Impulse on the particle due to disc:
Refer to the diagram.
pi→$\overrightarrow{p_i}$ and pf→$\overrightarrow{p_f}$ are the momentum of the particle before and after collision with the disc.
pi→=2mωRi^$\overrightarrow{p_i}=2m\omega R\hat{i}$
pf→=−m(ω3)Rj^$\overrightarrow{p_f}=-m({\displaystyle \frac{\omega }{3}})R\hat{j}$
Δp=pf→−pi→=−2mωRi^−m(ω3)Rj^=−mωR(2i^+13j^)$\mathrm{\Delta }p=\overrightarrow{p_f}-\overrightarrow{p_i}=-2m\omega R\hat{i}-m({\displaystyle \frac{\omega }{3}})R\hat{j}=-m\omega R(2\hat{i}+{\displaystyle \frac{1}{3}}\hat{j})$
Impulse

∣∣pf→−pi→∣∣=∣∣∣mωR(2i^+13j^)∣∣∣=mωR22+(13)2−−−−−−−−√=mωR37−−√3=37−−√3mωR$|\overrightarrow{p_f}-\overrightarrow{p_i}|=|m\omega R(2\hat{i}+{\displaystyle \frac{1}{3}}\hat{j})|=m\omega R\sqrt{2^2+({\displaystyle \frac{1}{3}}{)}^2}=m\omega R{\displaystyle \frac{\sqrt{37}}{3}}={\displaystyle \frac{\sqrt{37}}{3}}m\omega R$
Impulse
on the disc due to hinge will be balancing the impulse on the disc due
to particle which is  37−−√3mωR${\displaystyle \frac{\sqrt{37}}{3}}m\omega R$.

Definition

Condition of toppling

When a torque is applied on a body resisting change of motion (usually due to friction), the instantaneous axis of rotation shifts (usually due to shift of normal). The body topples if the instantaneous axis of rotation falls outside the body. This is shown in the attached diagram.

Example: A cubical block of mass m$m$ and side L$L$ rests on a rough horizontal surface with coefficient of friction μ$\mu$. A horizontal force F$F$ is applied on the block as shown in the figure. If the coefficient of friction is sufficiently high so that the block does not slide before toppling, find the minimum force required to topple the block.

Solution:
Considering case 1, when minimum force is applied,
F=μN$F=\mu N$
N=mg$N=mg$
∴F=μmg$\therefore F=\mu mg$
Now,
when the force exceeds some value, block topples
Taking ∑$\sum$ moment about the toppling axis,
∑M=0$\sum M=0$ ................ Still in equilibrium
∴∑M=∑F.d$\therefore \sum M=\sum F.d$
⇒0=F×L−mg×L2$\Rightarrow 0=F\times L-mg\times {\displaystyle \frac{L}{2}}$
∴F=mg2$\therefore F={\displaystyle \frac{mg}{2}}$

Example

Use instantaneous axis of rotation to find condition of total equilibrium

Example:
A uniform rod of length L rests against a smooth roller as shown in figure. Find the friction coefficient between the ground and the lower end if the minimum angle that rod makes with horizontal is θ$\theta$.

Solution:
Balance horizontal forces μN2=N1sinθ${\displaystyle \mu N_2=N_{1}sin\theta }$
Balance vertical force N1cosθ+N2=mg${\displaystyle N_{1}cos\theta +N_2=mg}$
or N1(cosθ+sinθμ)=mg${\displaystyle N_1(cos\theta +\frac{sin\theta }{\mu })=mg}$
or N1=μmgμcosθ+sinθ=mg${\displaystyle N_1=\frac{\mu mg}{\mu cos\theta +sin\theta }=mg}$
apply sinτ=0$sin\tau =0$ about point A.
N1sinθh+N1cosθhcotθ=mg12cosθ${\displaystyle N_{1}sin\theta h+N_{1}cos\theta hcot\theta =mg\frac{1}{2}cos\theta }$hN1[sin2θ+cos2θ]=mglcosθsinθ2${\displaystyle h{N}_1[si{n}^2\theta +co{s}^2\theta ]=\frac{mglcos\theta sin\theta }{2}}$hμmgμcosθ+sinθ=mgl2cosθsinθ${\displaystyle \frac{h\mu mg}{\mu cos\theta +sin\theta }=\frac{mgl}{2}cos\theta sin\theta }$2μh=μlcos2θsinθ+lcosθsin2θ${\displaystyle 2\mu h=\mu lco{s}^2\theta sin\theta +lcos\theta si{n}^2\theta }$μ(2h−lcos2θsinθ)=lcosθsin2θ${\displaystyle \mu (2h-lco{s}^2\theta sin\theta )=lcos\theta si{n}^2\theta }$μ=lcosθsin2θ2h−lcos2θsinθ${\displaystyle \mu =\frac{lcos\theta si{n}^2\theta }{2h-lco{s}^2\theta sin\theta }}$

Definition

Condition for toppling

When a torque is applied on a body resisting change of motion (usually due to friction), the instantaneous axis of rotation shifts (usually due to shift of normal). The body topples if the instantaneous axis of rotation falls outside the body. This is shown in the attached diagram.

Example

Solve Problem on instantaneous axis of rotation

Example: A rotating disc (shown above) moves in the positive direction of the x axis. Find the equation y(x)$y(x)$ describing the position of the instantaneous axis of rotation, if at the initial moment the axis C of the disc was located at the point O$O$ after which it moved with a constant velocity v$v$, while the disc started rotating counterclockwise with a constant angular acceleration β$\beta$ (the initial angular velocity is equal to zero),

Solution: The instantaneous velocity of the axis of rotation is zero with respect to outside frame
Here, y=R$y=R$
For disc, moving along x−$x-$axis, v=xtort=xv$v={\displaystyle \frac{x}{t}}ort={\displaystyle \frac{x}{v}}$ and for rotating w=vR=vy$w={\displaystyle \frac{v}{R}}={\displaystyle \frac{v}{y}}$  but, also w=βt$w=\beta t$
so, vy=βtorvy=βxvorv2βx=y

BookMarks

Page 1  Page 2  Page 3  Page 4  Page 5  Page 6  Page 7  Page 8  Page 9  Page 10
Page 11  Page 12