Rotational Dynamics Concepts Page - 11

Example

Velocity of the ring rolling down the inclined plane

Example: A circular ring starts rolling down on an inclined plane from its top.
Let V$V$ be velocity of its centre of mass on reaching the bottom of inclined plane. If a block starts sliding down on an identical inclined plane but smooth, from its top, then what is the velocity of block on reaching the bottom of inclined plane?

Solution: For ring
Work Energy Theorem
mgh=12mV2+12mR2(VR)2$mgh={\displaystyle \frac{1}{2}}m{V}^2+{\displaystyle \frac{1}{2}}m{R}^2{\left({\displaystyle \frac{V}{R}}\right)}^2$
mgh=mV2$mgh=m{V}^2$
V=gh−−√$V=\sqrt{gh}$
for a block
Work Energy Theorem
mgh=12mV21$mgh={\displaystyle \frac{1}{2}}m{V}_1^2$
V1=2gh−−−√$V_1=\sqrt{2gh}$
=2√V$=\sqrt{2}V$

Example

Velocity of disc rolling down the inclined plane

Example: A thin metal disc of radius 0.25m$0.25m$ and mass 2kg$2kg$ starts from rest and rolls down an inclined plane. If its rotational kinetic energy is 4J$4J$ at the foot of the inclined plane, then what is its linear velocity at the same point?
Solution:KER=12Iω2=4J$K{E}_R={\displaystyle \frac{1}{2}}I{\omega }^2=4J$
12×12mr2(vr)2=4J${\displaystyle \frac{1}{2}\times \frac{1}{2}m{r}^2{\left(\frac{v}{r}\right)}^2=4J}$
14mv2=4J${\displaystyle \frac{1}{4}}m{v}^2=4J$
v2=4×2×22$v^2={\displaystyle \frac{4\times 2\times 2}{2}}$
v=22√=2.82m/s$v=2\sqrt{2}=2.82m/s$

Example

Velocity of sphere rolling down the inclined plane

Example: A solid sphere of mass 0.1 Kg and radius 2 cm rolls down an inclined plane 1.4 m in length (slope 1 in 10). Starting from rest what will be its final
velocity ?

Solution:
We have tanθ=110$tan\theta ={\displaystyle \frac{1}{10}}$
or
θ=0.099$\theta =0.099$
or
sinθ=0.099$sin\theta =0.099$
Thus height of the of incline is given as 0.099×1.4=0.14m$0.099\times 1.4=0.14m$
Using conservation of energy we have
mg(0.14)=12mv2+15mv2$mg(0.14)={\displaystyle \frac{1}{2}m{v}^2+{\displaystyle \frac{1}{5}m{v}^2}}$
or
0.14g=710v2$0.14g={\displaystyle \frac{7}{10}{v}^2}$
or
v=0.14×9.81×107−−−−−−−−−−−−−−√$v=\sqrt{{\displaystyle {\displaystyle \frac{0.14\times 9.81\times 10}{7}}}}$
or
v=1.4 m/s$v=1.4m/s$

Example

Problems on rolling where more than one force contributes to torque

Example: A solid cylinder of mass 10kg$10kg$ is rolling perfectly on a plane of inclination 300${30}^0$. What is the force of friction between the cylinder and
surface of the inclined plane?
Solution:
Using Newton's 2nd Law of motion
Mgsinθ−f=Ma$Mg\sin \theta -f=Ma$  ......(1) along the inclined plane where f is the frictional force up the incline
Mgcosθ=N$Mg\cos \theta =N$      .........(2) perpendicular to the inclined plane
Substituting (2) in (1) we get,
Mgsinθ−μN=Ma$Mg\sin \theta -\mu N=Ma$
∴Mgsinθ−μMgcosθ=Ma$\therefore Mg\sin \theta -\mu Mg\cos \theta =Ma$
Only
frictional force exerts torque since weight mg$mg$ and normal reaction N$N$ pass through the center of the cylinder and do  not exert any torque. Torque due to frictional force is τ=fR$\tau =fR$ where R is the radius of the cylinder. Using equation for rotational motion,
τ=fR=Iα$\tau =fR=I\alpha$    .....(3) where I$I$ is the MI$MI$ of the cylinder about its
center and α$\alpha$ is the angular acceleration.
Since there is no slipping, linear acceleration a=Rα$a=R\alpha$
Substituting a=Rα$a=R\alpha$ in (3) we get,
f=IαR=12MR2×aR2=Ma2$f={\displaystyle \frac{I\alpha }{R}}={\displaystyle \frac{1}{2}}M{R}^2\times {\displaystyle \frac{a}{R^2}}={\displaystyle \frac{Ma}{2}}$    .....(4)
Substituting for Ma$Ma$ from eqn(1) in eqn(4)
f=Mgsinθ−f2$f={\displaystyle \frac{Mg\sin \theta -f}{2}}$
⇒3f=Mgsinθ$\Rightarrow 3f=Mg\sin \theta$
⇒f=Mgsinθ3=10×9.8×sin3003=493N$\Rightarrow f={\displaystyle \frac{Mg\sin \theta }{3}}={\displaystyle \frac{10\times 9.8\times \sin {30}^0}{3}}={\displaystyle \frac{49}{3}}N$

Example

Pure rolling using angular momentun conservation about point of contact

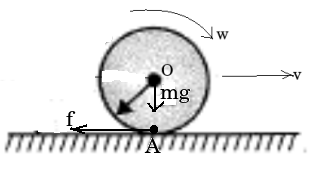
Example: A solid homogeneous sphere is moving on a rough horizontal surface, What will happen to the angular momentum about the point of contact during this kind of motion of sphere?

Solution: Net torque about the point A is Zero.Now,  dLAdt=τA=0${\displaystyle \frac{d{L}_A}{dt}}={\tau }_A=0$
Thus LA$L_A$ is conserved.

Example

Energy conservation in pure rolling

Example: A horizontal disc rotates freely about a vertical axis through its centre. A ring, having the same mass and radius as the disc, is now gently placed on the disc. After some time, the two rotate with a common angular velocity. What will be the further scenario in the motion?
Solution:In the question it is mentioned that finally both have same angular velocity and didn't mention about any external force  ,
   ⇒$\Rightarrow$ there exists friction between those two bodies .
  As friction is an internal force , Angular momentum about COM axis is conserved.
  mr22ω=mr22ω22+mr2ω22${\displaystyle \frac{m{r}^2}{2}}\omega ={\displaystyle \frac{m{r}^2}{2}}{\omega }_2^2+m{r}^2{\omega }_2^2$ {let ω2${\omega }_2$ be final angular velocity of
system.}
  ⇒ω2=ω3$\Rightarrow {\omega }_2={\displaystyle \frac{\omega }{3}}$
    Energy converted into heat=intial energy of system - final energy of system
  ⇒$\Rightarrow$Energy converted to heat = 12I1ω2−12I2ω22${\displaystyle \frac{1}{2}}{I}_1{\omega }^2-{\displaystyle \frac{1}{2}}{I}_2{\omega }_2^2$
Energy converted to heat=13mr2ω2=23${\displaystyle \frac{1}{3}}m{r}^2{\omega }^2={\displaystyle \frac{2}{3}}$ of intial kinetic energy.

Example

Impulsive Hinge Force

Example: A rigid equilateral triangular frame made of three identical thin rods (mass = m$m$ & length = l$l$) is free to rotate smoothly in vertical plane. The frame is hinged at one of its vertices H$H$. The frame is released from rest from the position shown in the figure. Find the hinge reaction?

Solution:
The individual weights each of mg$mg$  act downward at points x,y$x,y$ and z$z$ as shown in the figure.

The moment of each force mg about point H is given as
MX=mgl2$M_X=mg{\displaystyle \frac{l}{2}}$

MY=mgl2sin300$M_Y=mg{\displaystyle \frac{l}{2}}\sin {30}^0$

MZ=mg3√2lcos300$M_Z=mg{\displaystyle \frac{\sqrt{3}}{2}}l\cos {30}^0$
All the three torques exerted by the weights are in clockwise direction about point H.
τtotal=mg(l2+l2sin300+3√2lcos300=mgl(12+12×12+3√2×3√2)=32mgl${\displaystyle {\tau }_{total}=mg({\displaystyle \frac{l}{2}}+\frac{l}{2}\sin {30}^0+\frac{\sqrt{3}}{2}l\cos {30}^0=mgl(\frac{1}{2}+\frac{1}{2}\times \frac{1}{2}+{\displaystyle \frac{\sqrt{3}}{2}}\times \frac{\sqrt{3}}{2})={\displaystyle \frac{3}{2}}mgl}$

MI IHA=ml23$I_{HA}={\displaystyle \frac{m{l}^2}{3}}$

MI IHB=ml23$I_{HB}={\displaystyle \frac{m{l}^2}{3}}$

MI IAB=ml212+m(3√2l)2=5ml26$I_{AB}={\displaystyle \frac{m{l}^2}{12}}+m({\displaystyle \frac{\sqrt{3}}{2}}l{)}^2={\displaystyle \frac{5m{l}^2}{6}}$

Itotal=IHA+IHB+IAB=ml23+ml23+5ml26=32ml2$I_{total}=I_{HA}+I_{HB}+I_{AB}={\displaystyle \frac{m{l}^2}{3}}+{\displaystyle \frac{m{l}^2}{3}}+{\displaystyle \frac{5m{l}^2}{6}}={\displaystyle \frac{3}{2}}m{l}^2$

Equating the total torque τtotal${\tau }_{total}$ to Itotalω$I_{total}\omega$

32mgl=32ml2ω${\displaystyle \frac{3}{2}}mgl={\displaystyle \frac{3}{2}}m{l}^2\omega$

⇒ω=gl$\Rightarrow \omega ={\displaystyle \frac{g}{l}}$

Now for the hinge force we have
N1=3mgcos60∘=32mg${\displaystyle N_1=3mg\cos {60}^{\circ }=\frac{3}{2}mg}$
3mgsin60∘−N2=3m×gl×l3√⇒N2${\displaystyle 3mg\sin {60}^{\circ }-N_2=3m\times {\displaystyle \frac{g}{l}}\times {\displaystyle \frac{l}{\sqrt{3}}}\Rightarrow N_2}$=3√2mg${\displaystyle ={\displaystyle \frac{\sqrt{3}}{2}}mg}$

Hinge Force =N21+N22−−−−−−−−√=3√mg${\displaystyle =\sqrt{N_1^2+N_2^2}=\sqrt{3}mg}$

Definition

Angular Impulse

Definition: Angular momentum is a vector that is parallel to the angular velocity. If there is no net torque acting on a system, the system's angular momentum is conserved. A net torque produces a change in angular momentum that is equal to the torque multiplied by the time interval over which the torque is applied, which is a measurement of angular impulse.

BookMarks

Page 1  Page 2  Page 3  Page 4  Page 5  Page 6  Page 7  Page 8  Page 9  Page 10
Page 11  Page 12