Optics Concept Page - 19

Definition

Magnification of spherical lenses

Definition:

Magnification of a lens is defined as the ratio of the height of image to the height of object. It is also given in terms of image distance and object distance. It is equal to the ratio of image distance to that of  object distance.

m=hiho=vu$m={\displaystyle \frac{h_i}{h_o}}={\displaystyle \frac{v}{u}}$

Where, m= magnification

hi$h_i$ = height of image

ho$h_o$= height of object

Example

Problem on Magnification by spherical lenses

Problem: 
A object is placed at 30 cm on the principle axis of the convex lens from the lens and an image is formed 60 cm from the lens. If focal length of the lens is 20 cm then calculate the magnification.
Solution: 
Given :    u=−30$u=-30$  cmThe image is formed behind the lens as the object distance is greater than the focal length of the lens.⟹$⟹$     v=+60$v=+60$ cm∴$\therefore$ Magnification     m=−vu=−(60)−30=2.0$m={\displaystyle \frac{-v}{u}}={\displaystyle \frac{-(60)}{-30}}=2.0$

Definition

Power

Power of a lens is defined as the inverse of focal length (in meters) of the lens. It is a measure of the amount of deviation of light ray produced by a lens, more the power, more is the deviation. Unit of power is Dioptre (D$D$).
P=1f$P={\displaystyle \frac{1}{f}}$
Note:
Convex lens has positive power and concave lens has negative power.
Power of a plane glass plate is 0$0$.
Power of combinations of lenses kept close to each other is equal to the sum of individual powers of each lens.

Example

Calculate power of lens

Problem:
Magnification is 0.5 when the object distance is 10 cm. Find the power of the concave lens :
Solution:
m=vu=0.5$m={\displaystyle \frac{v}{u}}=0.5$

v10=0.5${\displaystyle \frac{v}{10}}=0.5$
v=5cm$v=5cm$
Now using:
1v−1u=1f${\displaystyle \frac{1}{v}}-{\displaystyle \frac{1}{u}}={\displaystyle \frac{1}{f}}$
1−5−1−10=1f${\displaystyle \frac{1}{-5}}-{\displaystyle \frac{1}{-10}}={\displaystyle \frac{1}{f}}$
f=−10cm$f=-10cm$
p=100−10 D$p={\displaystyle \frac{100}{-10}}D$
=−10D$=-10D$

Definition

Lens Power

Definition:

Whenever a ray of light passes through a lens (except when it passes through the optical center) it bends. The bending of light rays towards the principal axis is called convergence and bending of light rays away from the principal axis is called divergence. The degree of convergence or divergence of a lens is expressed in terms of its power. A lens of short focal length deviates the rays more while a lens of large focal length deviates the rays less. Thus power of a lens is defined as the reciprocal of its focal length in meters.

power of lens=1focal length$poweroflens={\displaystyle \frac{1}{focallength}}$
The unit of lens power is dioptre.

Example

Calculate lens power

Problem:
 The distance between an object and the screen is 100 cm.  A lens produces an image on the screen when placed at either positions 40 cm apart.  The power of the lens is

Solution:

For the first case we have
1v−1u=1f${\displaystyle \frac{1}{v}}-{\displaystyle \frac{1}{u}}={\displaystyle \frac{1}{f}}$
for second case we get
1100−u−(−1u)=1f${\displaystyle \frac{1}{100-u}}-(-{\displaystyle \frac{1}{u}})={\displaystyle \frac{1}{f}}$
Combining and solving
160−u−(−1u+40)=1f${\displaystyle \frac{1}{60-u}}-(-{\displaystyle \frac{1}{u+40}})={\displaystyle \frac{1}{f}}$

100(u)(100−u)=100(60−u)(u+40)${\displaystyle \frac{100}{\left(u\right)(100-u)}}={\displaystyle \frac{100}{(60-u)(u+40)}}$
240080=u${\displaystyle \frac{2400}{80}}=u$
u=30cm$u=30cm$
1f=170+130${\displaystyle \frac{1}{f}}={\displaystyle \frac{1}{70}}+{\displaystyle \frac{1}{30}}$
1f=100002100=${\displaystyle \frac{1}{f}}={\displaystyle \frac{10000}{2100}}=$ Power in D (cm is converted to m)
P≃5D$P\simeq 5D$

Formula

Combination of thin lenses in contact

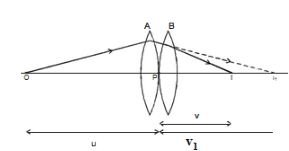
Consider two lenses A and B of focal length f1$f_1$ and f2$f_2$ placed in contact with each other.
An object is placed at O on  the common principal axis. The lens A produces an image at I1$I_1$ and this image acts as the object for the second lens B. The final image is produced at I$I$ as shown in figure.
PO = u, object distance for the first lens (A),
PI = v, final image distance and
PI1=v1$P{I}_1=v_1$, image distance for the first lens (A) and also object distance for second lens (B).
For the image I1$I_1$ produced by the first lens A,
1v1−1u=1f1${\displaystyle \frac{1}{v_1}}-{\displaystyle \frac{1}{u}}={\displaystyle \frac{1}{f_1}}$  .... (1)
For the final image I, produced by the second lens B,
1v−1v1=1f2${\displaystyle \frac{1}{v}}-{\displaystyle \frac{1}{v_1}}={\displaystyle \frac{1}{f_2}}$  ... (2)
Adding equations (1) and (2),
1v−1u=1f1+1f2${\displaystyle \frac{1}{v}}-{\displaystyle \frac{1}{u}}={\displaystyle \frac{1}{f_1}}+{\displaystyle \frac{1}{f_2}}$   ... (3)
If the combination is replaced by a single lens of focal length F such that it forms the image of O at the same position I, then
1v−1u=1F${\displaystyle \frac{1}{v}}-{\displaystyle \frac{1}{u}}={\displaystyle \frac{1}{F}}$ ... (4)
From equations (3) and (4),
1F=1f1+1f2${\displaystyle \frac{1}{F}}={\displaystyle \frac{1}{f_1}}+{\displaystyle \frac{1}{f_2}}$...... (5)
This F is the focal length of the equivalent lens for the combination.

Example

Effective focal length of thin lenses placed in contact

Three lenses in contact have a combined focal length of 12cm$12cm$. When the third lens is removed the combined focal length becomes 607cm${\displaystyle \frac{60}{7}}cm$. What is the focal length of the third lens?
Let f1$f_1$, f2$f_2$ and f3$f_3$ be three focal lengths of the lenses.
Combined Focal length: f=12 cm$f=12cm$
So, 1f=1f1+1f2+1f3${\displaystyle \frac{1}{f}}={\displaystyle \frac{1}{f_1}}+{\displaystyle \frac{1}{f_2}}+{\displaystyle \frac{1}{f_3}}$
When the third lens is removed, 1f′′=1f1+1f2${\displaystyle \frac{1}{f^{″}}}={\displaystyle \frac{1}{f_1}}+{\displaystyle \frac{1}{f_2}}$, where f′′=607$f^{″}={\displaystyle \frac{60}{7}}$
This means, 112=1607+1f3${\displaystyle \frac{1}{12}}={\displaystyle \frac{1}{{\displaystyle \frac{60}{7}}}}+{\displaystyle \frac{1}{f_3}}$
⇒ f3=−30 cm$\Rightarrow f_3=-30cm$
Since f3$f_3$ is negative, it is a diverging lens having focal length 30 cm$30cm$.

BookMarks

Page 1  Page 2  Page 3  Page 4  Page 5  Page 6  Page 7  Page 8  Page 9  Page 10  Page 11
Page 12  Page 13  Page 14  Page 15  Page 16  Page 17  Page 18  Page 19  Page 20  Page 21 
Page 22  Page 23  Page 24