Centre of Mass, Momentum and Collision Concepts Page - 8

Example

Collisions combined with pulley mass system

Example:
P and Q are two identical masses at rest suspended by an inextensible string passing over a smooth frictionless pulley. Mass P is given a downward push with a speed v as shown in figure. It collides elastically with the floor and rebounds immediately. What happens immediately after collision?
Solution:
Just after collision, P reverses its direction of motion causing the string to become loose. This results in zero tension in the wire. Q continues to move upward with velocity v due to inertia.

Example

Collisions with pulley mass system with tension as impulsive force

Example:
A block of mass m$m$ and a pan of the same mass are connected by a pulley as shown in the figure. A particle of mass m$m$ travelling with a velocity v$v$ collides and stick to the pan as shown. Find the velocity of blocks after collision if initially the pan is at rest.
Solution: 
Let N$N$ be the contact force between the particle and the pan and V$V$ be the final velocity. Impulse imparted equals the change in momentum.
For particle, Ndt=mv−mV$Ndt=mv-mV$
For pan, (N−T)dt=mV$(N-T)dt=mV$
For block, Tdt=mV$Tdt=mV$
Solving, V=v3$V={\displaystyle \frac{v}{3}}$

Example

Conservation of Angular Momentum for collision of rigid bodies

Example: A spherical ball of mass M$M$ moving with initial velocity v$v$ collides elastically with another ball of mass M$M$, which is fixed at one end of L$L$ shaped rigid massless frame as shown in figure (a). The L$L$ shaped frame contains another mass M$M$ connected at the other end. What is the speed of the striking mass after collision?

Solution: Consider the L to be a single system. Its COM lies in midway of the diagonal.
The initial velocity of ball be u$u$ and final velocity (in same direction) be v$v$.
Linear momentum is always conserved. Momentum imparted to the L-system after collision is = m(u−v)=2m(vLcom)$m(u-v)=2m(v_{Lcom})$
vLcom=(u−v)/2$v_{Lcom}=(u-v)/2$
About COM of L-system(just consider an axis about it) angular momentum of the 3 body system is conserved.
Li=mu(l/2)$L_i=mu(l/2)$. Lf=mv(l/2)+2m(vcom)(0)+ILωL$L_f=mv(l/2)+2m(v_{com})(0)+I_L{\omega }_L$
IL=2×m(l/2√)2=ml2$I_L=2\times m(l/\sqrt{2}{)}^2=m{l}^2$
=>(u−v)/2=lω$=>(u-v)/2=l\omega$
velocity of separation of the balls is vLcom+(l/2)ω−v$v_{Lcom}+(l/2)\omega -v$
lω/2$l\omega /2$ is horizontal component of velocity obtained by angular motion.
In elastic collision, velocity of approach = velocity of separation.
Therefore, u=vLcom+(u−v)/4−v=3(u−4)/4−v$u=v_{Lcom}+(u-v)/4-v=3(u-4)/4-v$
4(u+v)=3(u−v)=>v=−u/7$4(u+v)=3(u-v)=>v=-u/7$

Example

Coefficient of restitution in collisions involving rigid bodies

Example: A uniform vertical rod of mass m$m$ and length 2l$2l$ is hinged at its highest point and is initially at rest. A small pendulum of mass m and string length l$l$, is also suspended from the same point. Initially it is at an angle 600${}^0$ with vertical and then it is released. The pendulum strikes the rod, just after collision, the pendulum comes to rest, the angular speed of the rod becomes ω$\omega$ and coefficient of restitution of this collision is e$e$, then, find e$e$ and angular velocity.

Solution: The ball will strike the rod with a velocity of
v=2gR(1−cosθ)−−−−−−−−−−−−√⇒v=gl−√${\displaystyle v=\sqrt{2gR(1-\cos \theta )}\Rightarrow v=\sqrt{gl}}$
Applying angular momentum conservation about the hinge point

m(gl−√)l+0=0+(m(2l)23)(ω)$m\left(\sqrt{gl}\right)l+0=0+\left({\displaystyle \frac{m{\left(2l\right)}^2}{3}}\right)\left(\omega \right)$     get

ω=34gl−−√${\displaystyle \omega ={\displaystyle \frac{3}{4}}\sqrt{{\displaystyle \frac{g}{l}}}}$ 

also e=VelocityofseparationaftercollisionVelocityofapproachbeforecollision${\displaystyle e={\displaystyle \frac{Velocityofseparationaftercollision}{Velocityofapproachbeforecollision}}}$
⇒e=0+ωlgl−√${\displaystyle \Rightarrow e=\frac{0+\omega l}{\sqrt{gl}}}$     where ω=34gl−−√$\omega ={\displaystyle \frac{3}{4}}\sqrt{{\displaystyle \frac{g}{l}}}$
So e=34${\displaystyle e={\displaystyle \frac{3}{4}}}$

Example

Problems on inelastic collision of point masses with rigid bodies rotating about fixed axis

Example: A uniform rod of length L and mass M is lying on a frictionless horizontal plane and is pivoted at one of its ends as shown in Fig. There is no friction at the pivot. An inelastic ball of mass m is fixed with the rod at a distance L/3 from O. A horizontal impulse J is given to the rod at a distance 2L/3 from O in a direction perpendicular to the rod. Assume that the ball remains in contact with the rod after the collision and impulse J acts for a small time interval Δt$\mathrm{\Delta }t$. Find the resulting instantaneous angular velocity of the rod after the impulse.
Solution:
Let the system starts with angular velocity ω$\omega$. Angular velocity

of the ball will also be ω$\omega$ as it remains struck to the rod.
Velocity of the ball v=ωL3$v=\omega {\displaystyle \frac{L}{3}}$
For the rod, angular impulse =$=$ change in angular momentum:
J2L3−JbL3=ML23ω$J{\displaystyle \frac{2L}{3}}-J_b{\displaystyle \frac{L}{3}}={\displaystyle \frac{M{L}^2}{3}}\omega$ ....(i)
For the ball, impulse =$=$ change in linear momentum
Jb=mv=mω(L3)$J_b=mv=m\omega \left({\displaystyle \frac{L}{3}}\right)$ ....(ii)
From Eqs. (i) and (ii), ω=6J(m+3m)L$\omega ={\displaystyle \frac{6J}{(m+3m)L}}$ and Jb=2mJ(m3M)$J_b={\displaystyle \frac{2mJ}{(m_{3}M)}}$
Let impulse on the pivot be J1$J_1$
For the rod and ball system, total impulse =$=$ change in linear momentum: J+J1=Mvc+mv$J+J_1=M{v}_c+mv$
Solve to get: J1=mJm+3M$J_1={\displaystyle \frac{mJ}{m+3M}}$

Example

Elastic collision of point masses with rigid bodies rotating about fixed axis

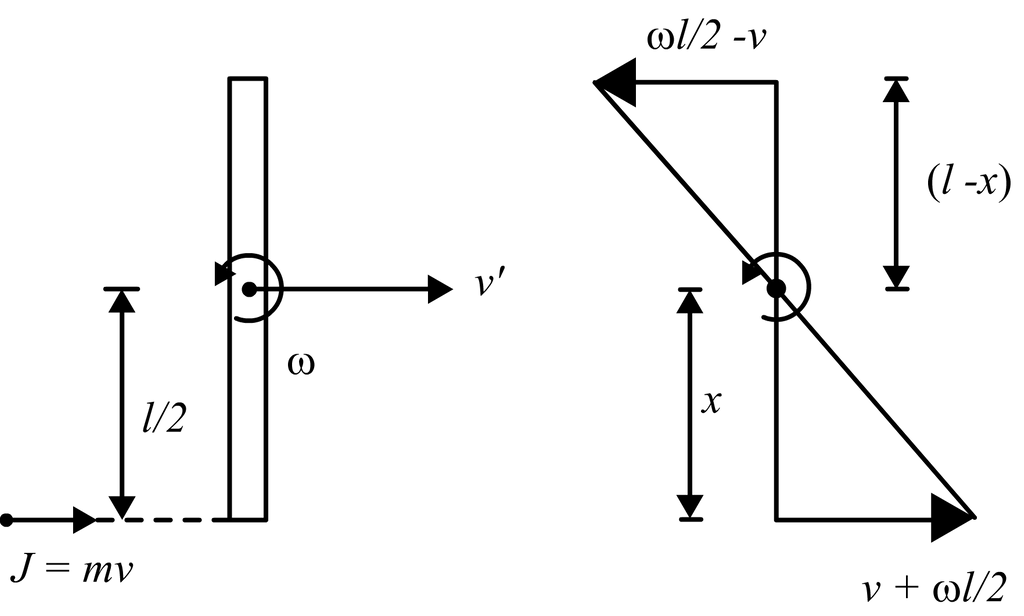
Example: An impulse J=mv$J=mv$ at one end of a stationary uniform frictionless rod

of mass m$m$ and length l$l$ which is free to rotate in a gravity-free

space. The impact is elastic. Instantaneous axis of rotation of the rod

will pass through which place?

Solution:J=mv$J=mv$
⇒$\Rightarrow$ Velocity of the CM of rod =v$=v$
Applying impulse momentum equation about the CM of rod
Jl2=ICMω⇒mvl2=(ml212)ω⇒ω=6vl$J{\displaystyle \frac{l}{2}}=I_{CM}\omega \Rightarrow {\displaystyle \frac{mvl}{2}}=\left({\displaystyle \frac{m{l}^2}{12}}\right)\omega \Rightarrow \omega ={\displaystyle \frac{6v}{l}}$
About instantaneous axis of rotation the rod is considered to have pure rotation.
Let instantaneous axis of rotation be located at a distance x$x$ from the colliding end [Fig (b)]
ωl2−v1−x=v+ωl2x${\displaystyle {\displaystyle \frac{{\displaystyle \frac{\omega l}{2}}-v}{1-x}}={\displaystyle \frac{v+{\displaystyle \frac{\omega l}{2}}}{x}}}$ ....(i)
Substituting the value of ω=6v/l$\omega =6v/l$ in Eq. (i), we get x=23l$x={\displaystyle \frac{2}{3}}l$.

Example

HInge force on rigid bodies

Example: A thin uniform square plate with side l$l$ and mass M$M$ can rotate freely about a stationary vertical axis coinciding with one of its sides. A small ball of mass m$m$ flying with velocity v⃗ $\overrightarrow{v}$ at right angles to the plate strikes elastically the centre of it. Find the horizontal component of the resultant force which the axis will exert on the plate after the impact.

Solution: Angular momentum conservation:
l2m(v−v′)=Ml23w${\displaystyle \frac{l}{2}}m(v-v^{{}^{\prime }})={\displaystyle \frac{M{l}^2}{3}}w$
From above solution,
v′=3m−4M3m+4Mv$v^{{}^{\prime }}={\displaystyle \frac{3m-4M}{3m+4M}}v$
Substituting in momentum equation, we get,
w=4vl(1+4M3m)$w={\displaystyle \frac{4v}{l(1+{\displaystyle \frac{4M}{3m}})}}$

Horizontal reaction force acting on the plate will be the centripetal force given by,
dF=Mdxlw2x$dF={\displaystyle \frac{Mdx}{l}}{w}^{2}x$
F=Ml2w2$F={\displaystyle \frac{Ml}{2}}{w}^2$
F=8Mv2l(1+4M3m)2

BookMarks

Page 1  Page 2  Page 3  Page 4  Page 5  Page 6  Page 7  Page 8  Page 9